Algebra Archives

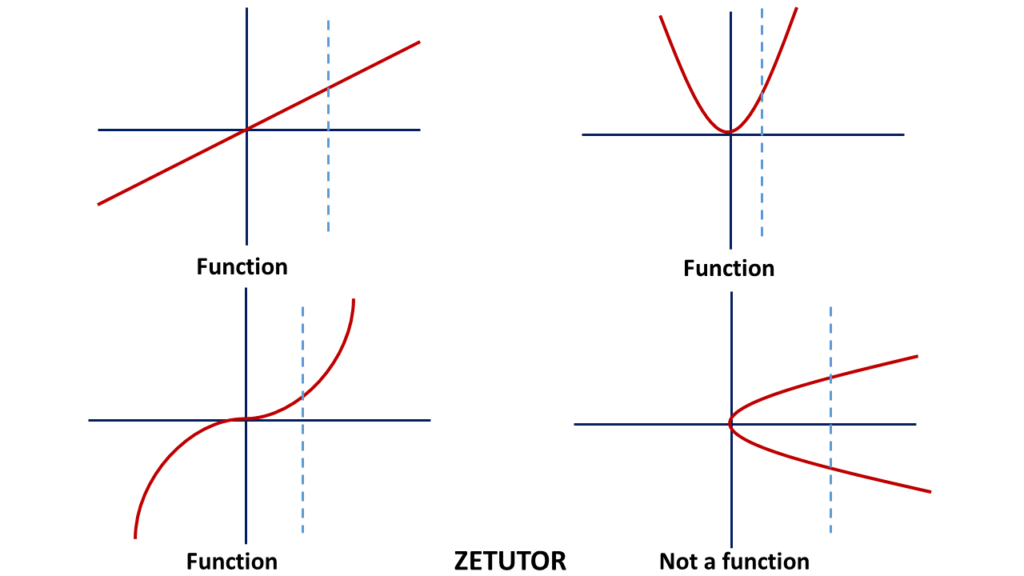
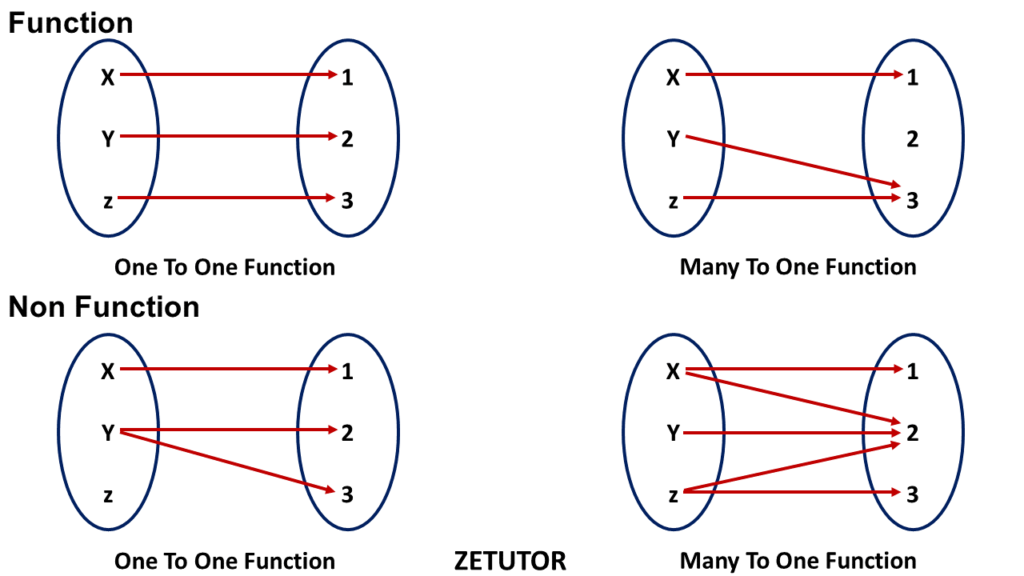
Linear Function

Leave a Comment / Algebra / By zetutor.com

LINEAR FUNCTION

Linear function has a linear relationship between x variable (independent variable) and y variable (dependent variable). This implies a constant rate of change.

For every unit increase in the x variable, the dependent variable y changes with a constant amount. The The general form of linear function is given by

f(x) = mx + b

where,

x input or independent variable

f(x) output or dependent variable

m is the slope of the line

b is the y intercept

SLOPE INTERCEPT FORM

The slope intercept form represents the equation of a straight line. The slope intercept form is given by

y = mx + b

where,

y – Dependent variable

x – Independent variable

m – Slope

b – Y Intercept (y-axis crossing point)

Example: y = 5x + 7 is a slope intercept form

slope = 5, y intercept = 7

SLOPE

The slope is the rate of change or simply it represents the steepness of the line. The slope is the coefficient of the independent variable x or the number in front of the x. The slope indicates the y changes for a unit change in x.

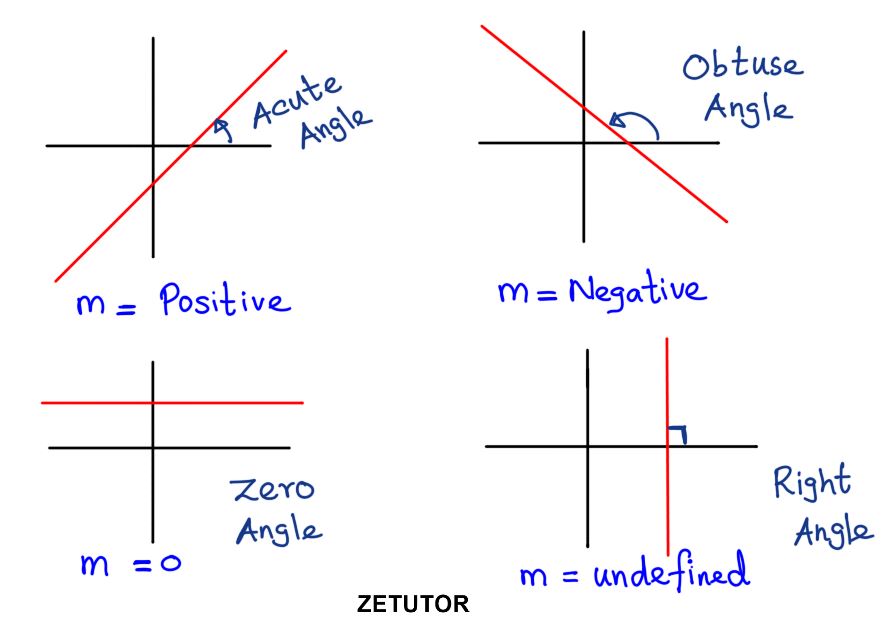
According to the sign of the slope, the line’s angle with horizontal will change

m positive – Line makes an acute angle with horizontal

m negative – Line makes an obtuse angle with horizontal

m zero – Horizontal line

m undefined -Vertical line

The slope is used in mathematics and physics. In physics slope of a position vs time, graph is equal to velocity. In a velocity vs time graph, the slope is equal to acceleration.

SLOPE FORMULA

$slope =\frac{rise}{run}$

$slope =\frac{Change in y}{Change in x}$

$slope =\frac{y_{2}-y_{1}}{x_{2}-x_{1}}$

where

$(x_{1}, x_{1}) and (x_{2},y_{2})$ two coordinates

Check the video for examples

Y INTERCEPT

This is the point where the graph crosses the y axis. You can find y intercept by making x=0 in the y=mx+b equation. Always x coordinate of y intercept equals to zero. So the y intercept coordinate will be (0,b)

POINT SLOPE FORM EQUATION

Point Slope form is a another way to express the linear equations but it’s particularly used when you know the coordinates of a specific point on the line and the slope of the line. The point slope form equation is given by

y-y_{1}=m(x-x_{1})

$(x_{1},y_{1})$ known coordinate

m is the slope of the line

Plugin the known coordinate and slope in the point slope form equation and after simplifying that we are able to change point slope form equation to slope intercept form y=mx+b

EQUATION OF LINES

Point slope form $y-y_{1}=m(x-x_{1})$

Slope intercept form y = mx + b

Horizontal line x=0

Vertical line y=0

General form Ax+By+C=0

PARALLEL LINES

If two lines are not intersect and maintains a constant distance between each of them they are parallel line. Parallel lines have the same slope. For example if you consider the two parallel lines only the y intercept is different. So the two equations will be like this. y=mx+b and y=mx+c where b and c are the y intercepts

Parallel lines run side by side do not converge or diverge.

Example: Line y=3x+5 and y=3x+7 are parallel because both has same slope 3

PERPENDICULAR LINES

When two lines intersect with 90 degrees angle(right angle) they are perpendicular lines. The slopes of perpendicular lines are negative reciprocal of each other.

m_{1}\times ,m_{2}=-1

If the slope of the line is m, the slope of the perpendicular line will be -1/m

Example: y=2x+4 and $y=\frac{1}{2}x+8$ are perpendicular because $2\times\frac{1}{2}=-1$

EPILOGUE

Linear equations have many applications in physics, engineering, economics and biology. They provide a simple yet powerful tool for modeling and analyzing relationships between variables with a constant rate of change. The graphical representation of linear functions is straight line which is very helpful in both theoretical and practical contexts.

Completing The Square

Leave a Comment / Algebra / By zetutor.com

COMPLETING THE SQUARE

Completing the square method is used to convert the quadratic equation from general form $ax^2+bx+c$ to vertex form $a(x-h)^2 +k$ .

Application of completing the square method

1. Solving quadratic equation

2. Converting to vertex form

3. Graphing a quadratic function

Completing The Square Method Steps

Start with the quadratic equation
Divide through by the coefficient of $x^2$
Take half of the coefficient of and square it.
Add and subtract the squared term inside the parentheses.
Factor the perfect square trinomial and simplify
Combine like terms
Simplify further if possible

Application Of Completing The Square Methods

Completing the square method has various real world an mathematical application.

1. Solving quadratic equations

The main application of this method is to solve quadratic equation. Completing square method is a quadratic equation solver. If you want to solve the quadratic equation first you need to change the standard form of quadratic equation into a perfect square trinomial. After that by taking the square roots on both sides we can find the solution.

2. Graphing a quadratic equation

Using the complete square method we can change the standard form into a vertex form. Graphing a quadratic function is much simpler in the vertex form than in the standard form. $a(x-h)^2 +k$ where (h,k) is the vertex of parabola.

3.Optimization Problems

Maximum or Minimum values can be found using the completing square methods. To find the maximum and minimum standard form quadratic equation should be converted to vertex form. $a(x-h)^2 +k$ where (h,k) is the vertex of parabola.

4. Physics Equations

Completing the square method has various application in physics. Some kinematics or laws of motion equations can be in a quadratic equation. By changing to vertex form we can find the solutions

Functions

Leave a Comment / Algebra / By zetutor.com

FUNCTIONS

A function is a relation between a set of inputs (x values) and a set of possible outputs (y values).

A function is like a machine. If you give a input to a function it will give you an an output.

F(x) is the function notation. F(x) is not f times x, you need to read it as function of x

Domain : All the inputs are called domain

Range: All the outputs are called range.

Example: let’s take f(x) = x+3 is a function.

Let’s give an input x=6, so x=6 one of the domain. If you give input 6 you will get an output f(x) = 6+3=9. So f(x)= 9 is one of the range value.

IDENTIFYING FUNCTIONS

Not all the equations are functions. There are some methods used to identify the functions

1. Vertical Line Test

2. Mapping Of Elements

VERTICAL LINE TEST

For a relation to be function each input value ( x value) must be associated with only one output value (y value).

First draw the graph of a given function and draw a vertical line and moving it across the graph.

If at any point the vertical line intersects the graph at more than one point, the relation is not a function.

A relation is a function if the vertical line intersects the graph at most one point for each potential x-value.

MAPPING OF ELEMENTS

Let’s take input on one side and output on another side. One To One and Many To One is a function.

One To Many and Many To Many is not a function.

One To One Function

This is a function between two sets. Let’s take the two sets as A and B. If every element in A connects with a distinct element of B, then it is a One To One function. Set A is domain and set B is a codomain

Definition of a function also can be expressed using domain and codomain. When every element of domain set connects with a distinctive element of codomain set it will be considered as one to one function. This is also called as injective function.

Many To One Function

This is known as non injective function. This is a type of mathematical function where different element in set A connects with the same element in set B. In a many to one function distinct element in the domain set can map to the same element in the domain set.

If you draw a horizontal line to the many to one function graph, it will intersect the graph at two points.

Types of Functions

Types of function can be represented in many ways. Here is the list of common list.

1. Polynomial Function – Linear Function, Quadratic Function, Cubic Function, Quartic Function, Quintic Function

2. Trigonometric Function

3. Exponential Function

4. Logarithmic Function

5. Rational Function

6. Modulus Function

7. Inverse Function

8. Greatest Integer Function

Polynomial Function

A polynomial function is a algebraic function. Polynomial function contain variables of varying degrees, coefficients and constants. The standard of polynomial function is given below

f(x)=a_{n}x^{^{n}}+a_{n-1}x^{^{n-1}}+a_{2}x^{^{2}}+a_{1}x++a_{0}

Where,

n is the degree of the polynomial and non negative integer.

$a_{n}$ is the leading coefficient

$a_{n}, a_{n-1}, a_{0}$ are the coefficient of the variable
Degree is the highest exponent of a given polynomial. Coefficients can be real number or complex number.

Trigonometric Function

Trigonometric functions are mainly used for right triangles. It creates a connection between the side and angle of a triangle. There are 3 major trigonometric functions sine(sin), cosine(cos) and tangent(tan) and there are 3 minor trigonometric function cosecant(csc), secant(sec), cotangent(cot).

Exponential Function

$y=ab^x$ is the general form of an exponential function. where a, b are constants and x is an independent variable. $y=e^x$ is also considered as an exponential function but we have special name for that it’s called natural exponents. When a exponential function increases it is called exponential growth and when it decreases it is called exponential decay.

Logarithmic Function

Logarithmic functions are the inverse of exponential function. Logarithmic functions are denoted by $\log_{a}x$ . Here a is the base and x is the argument. We can classify the logarithm mainly into two types

Common Logarithm : These type of logarithm has a base of 10 and it is denoted by log x

Natural Logarithm : When the base is e (Euler’s number) it is called natural logarithm. Euler’s number is an irrational constant approximately equals to 2.71828…

Rational Function

Rational function can be expressed as a quotient or a fraction of two polynomial. So in simple words if you have polynomials in the numerator and denominator that function can be considered as a ration function.

The general form of rational function is given below.

y=\frac{f(x)}{g(x)}

y is a rational function and f(x), g(x) are polynomials. g(x) is not equal to 0 because when the denominator equals to zero the whole rational function will become undefined. Rational functions are mainly used to describe the ratios and proportions.

Quadratic Equation

Leave a Comment / Algebra / By zetutor.com

FACTORING QUADRATIC EQUATIONS

Quadratic equation is a second degree polynomial function with the single variable. The standard form quadratic function is given by

$ax^2+bx+c=0$ , where x represents the variable and a is the coefficent of $x^2$ , b is the coefficient of x and c is a constant. a,b,c can take all real numbers. a is not equal to zero because if a is equals to zero then the second degree polynomial won’t exist

The solution of the quadratic equation can be found using the quadratic formula

$x=\frac{-b\pm \sqrt{b^{^{2}}-4ac}}{2a}$

In the quadratic formula discriminant is an important one. The term inside the square root $b^2-4ac$ is called discriminant. Discriminant plays an important rule to determine the nature of the solutions. The three cases are given below

1. $b^2-4ac>0$ : This means discriminant is positive because greater than zero indicates the positive numbers. When the discriminant is positive, the quadratic equation have two distinct real solutions/roots/zeros. If you draw the graph of positive discriminant quadratic function the graph will intersect the x axis at two distinct points.

2. $b^2-4ac=0$ : This means discriminant equals to zero. When the discriminant is zero, there is exactly one real solution. These types of quadratic equations are perfect square trinomial. If you draw the graph of the discriminant zero quadratic function, it will intersect the x axis at exactly one point. This is a single point of intersection.

3. $b^2-4ac<0$ : This means discriminant is negative because less than zero indicates the negative numbers. When the discriminant is negative, the quadratic equation have two complex solution which involves imaginary numbers. And the graph won’t intersect the x axis at any point.

Factoring quadratic equations is a method of expressing them as a product of two linear functions.

Example: $x^2+5x+6 =(x+2)(x+3)$

(x+2) and (x+3) are the factors of $x^2+5x+6$

Factoring Quadratics Equations Methods

1. Cross Method / Product Sum Method

2. Algebraic Identities

3. Quadratic Formula

Cross Method / Product Sum Method

Consider a quadratic equation $x^2+6x+8 = 0$

1. Find two numbers m and n such that m x n = 8 (constant), m+n=6 (middle term)

2. According to the first step m=4, n=2

3. Rewrite the middle term using m and n

So the final answer is $x^2+6x+8 = (x+4)(x+2)$

Algebraic Identities

Algebraic identities are expressions that are valid for all values of the variable. There are different algebraic identities. Here are some of them.

1. Factorizing a perfect square trinomial

$a^2+2ab+b^2=(a+b)^2$

$a^2-2ab+b^2=(a-b)^2$

Example $x^2-10x+25=(x-5)^2$

2. Factorizing the difference of squares

$a^2-b^2=(a+b)(a-b)$

Example $x^2-4^2=(x+4)(x-4)$

3. Factorizing the sum of cubes

$a^3+b^3=(a+b)(a^2-ab+b^2)$

a^3-b^3=(a-b)(a^2+ab+b^2)

Example:

a^3+b^3=(a+b)(a^2-ab+b^2)

4. Factorizing the cubes of the sum

(a+b)^3=a^3+3a^2b+3ab^2+b^3

(a-b)^3=a^3-3a^2b+3ab^2-b^3

Example

(x+5)^3=x^3+15x^2+75x+125

(x-5)^3=x^3-15x^2+75x+125

Quadratic Formula

The quadratic formula is a direct method of finding the solution of a quadratic function. Above mentioned cross method or algebraic identities can not be used for all quadratic functions but the quadratic formula can be used for all quadratic functions. The quadratic formula is given below

x=\frac{-b\pm \sqrt{b^{^{2}}-4ac}}{2a}

Where,

x = unknown variable

a = coefficient of $x^2$ , b – coefficient of x, c – constant

$\pm$ = This means there is a possibility for two solutions. One with a Plus sign and another with a Minus sign.

Follow these steps to use the quadratic formula

1. Find the a, b, c values from the quadratic equation

2. Plugin the values in the quadratic formula

3. Simplify the equation and find the solutions.

Example: $2x^2-5x+2$

a=2, b=-5, c=2

$x=\frac{-(-5)\pm \sqrt{(-5)^{^{2}}-4(2)(2)}}{2(2)}$

x=\frac{5\pm \sqrt{25-16}}{4}

$x=\frac{5\pm \sqrt{9}}{4}$

x=\frac{5\pm 3}{4}

Plus $x=\frac{5+3}{4}$

$x=\frac{8}{4}$

x=2

Minus $x=\frac{5-3}{4}$

$x=\frac{2}{4}$

$x=\frac{1}{2}$

Not all quadratic equations are factorable over the real numbers. In this case quadratic formula is the only way to find the solution or factors.

Applications Of Factoring Quadratic Equation

1. Solving Equations

Factoring is one of the easiest and most effective ways to solve a quadratic equation.

2. Quadratic Graph Analyze

Factoring is used to find the x intercepts or roots and y intercepts

3. Algebraic Simplification

Most of the time algebraic expressions or equations are complex, factoring can be used to simplify the algebraic equations.